NAEST 2020 Acceleration of the Centre of Mass of a Falling S

Question: Neglect any effect of friction during the fall of this uniform scale. The center of mass of the scale

1. falls with a vertically downward component of acceleration equal to g.
2. falls with a vertically downward component of acceleration less than g.
3. falls with a vertically downward component of acceleration more than g.
4. moves with a speed half that of the farthest point of the scale at any instant during the fall.

Solution: The forces on the scale in the vertical direction are its weight $mg$ and the normal reaction $N$ from the floor at the contact point. The normal reaction is vertically upward and non zero. Thus, force on the scale in the vertical direction is $mg-N$. Apply Newton's second law in the vertical direction to get the vertical component of the acceleration of scale's centre of mass as $a = g-N/m < g$. Thus, option (B) is correct.

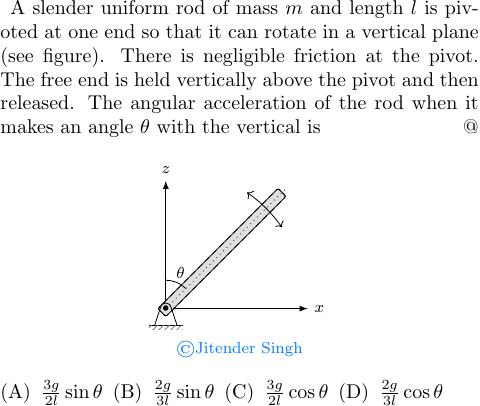
You may assume scale to be pivoted at the contact point. In this case, you can find an expression for its angular acceleration as $\alpha = (3g/2l)\sin\theta$, where $l$ is the scale's length and $\theta$ is the angle made by the scale with the vertical. The total acceleration of the scale's centre of mass is $a_t = \alpha l/2 = (3g/4)\sin\theta$ and the vertical component of the acceleration is $a=(3g/4)\sin^2\theta$.

Let $\omega$ be the angular velocity of the scale at some instant. The velocity of the centre of mass is $\omega l/2$ and the velocity of the end point is $\omega l$. Thus, option (D) is correct.

Related Question:

Solution to Rotational Mechanics of Falling Rod

See Our Books