Relative Velocity and River Boat Problem
To solve river boat problems, we need to understand two concepts:
- The speed of a boat relative to the water is equal to the speed of boat in still water.
- The velocity of a boat relative to the water ($\vec{v}_{b/w}$) is equal to the difference in velocity of the boat relative to the ground ($\vec{v}_b$) and velocity of water relative to the ground ($\vec{v}_w$) i.e., \begin{align} \vec{v}_{b/w}=\vec{v}_b-\vec{v}_w \nonumber \end{align}
Motion along the River
Downstream Motion
Problem: The velocity of a boat in still water is 10 km/hr and the velocity of river water is 5 km/hr. What is the time taken by the boat to travel a distance of 30 km downstream?
Solution: Let $x$ axis be in the downstream direction. Given, $\vec{v}_w=5\;\mathrm{km/hr}\;\hat\imath$ and $\vec{v}_{b/w}=10\;\mathrm{km/hr}\;\hat\imath$. The velocity of boat relative to the ground is given by relative velocity formula $\vec{v}_b=\vec{v}_{b/w}+\vec{v}_w=15\;\mathrm{km/hr}\:\hat\imath$. Thus, time taken by the boat to travel a distance $d=30\;\mathrm{km}$ is \begin{align} t=\frac{d}{|\vec{v}_b|}=\frac{30}{15}=2\;\mathrm{hr}\nonumber \end{align} In downstream motion, the speed of the boat is equal to the sum of the speed of boat in still water and the speed of river water.
Upstream Motion
Problem: What is the time taken by the boat if it travels same distance upstream?
Solution:
In this case, $\vec{v}_w=5\;\mathrm{km/hr}\;\hat\imath$, $\vec{v}_{b/w}=-10\;\mathrm{km/hr}\;\hat\imath$, and $\vec{v}_b=\vec{v}_{b/w}+\vec{v}_w=-5\;\mathrm{km/hr}\:\hat\imath$. The time taken by the boat $t={d}/{|\vec{v}_b|}={30}/{5}=6\;\mathrm{hr}$.
In upstream motion, the speed of the boat is equal to the difference of the speed of boat in still water and the speed of river water.
Problem: A motorboat going downstream overcame a raft at a point A; one hour later it turned back and after some time passed the raft at a distance 6.0 km from the point A. Find the flow velocity.
Solution: The speed of the raft is same as speed of the flow, say $v_r$. Let $v_b$ be speed of the boat in still water. The boat travels for 1 hr downstream with a speed $v_b+v_r$. The distance traveled by the boat in its downstream journey from A to its turning point C is \begin{align} \mathrm{AC}=(v_b+v_r)(1)=v_b+v_r. \end{align} In its return journey, the boat meets the raft at a point B. The speed of the boat in its return journey (upstream) is $v_b-v_r$. If the boat takes time $t$ hour from C to B then the distance BC is \begin{align} \mathrm{BC}=(v_b-v_r)t.\nonumber \end{align} The raft travels from A to B with a speed $v_r$ for time $(1+t)$ hour i.e., \begin{align} \mathrm{AB}=v_r (1+t).\nonumber \end{align} Now, $\mathrm{AB}=\mathrm{AC}-\mathrm{BC}=6\;\mathrm{km}$. Substitute expressions for AC, BC, and AB and solve to get flow speed $v_r=3\;\mathrm{km/hr}$.
Motion across the River
Shortest Distance
Problem: (IIT JEE 1988) A boat, which has a speed of 5 km/hr in still water, crosses a river of width 1 km along the shortest possible path in 15 minutes. The velocity of the river water is?
Solution: The boat crosses the river by the shortest path if it moves perpendicular to the river current.
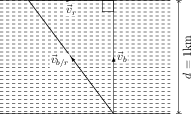
Question: You cannot cross a river to an exactly opposite point if your speed in still water is less than the speed of river water.
Minimum Time
Problem: (IIT JEE 1983) A river is flowing from west to east at a speed of 5 metre/minute. A man on the south bank of the river, capable of swimming at 10 metre/minute in still water, wants to swim across the river in the shortest time. He should swim in a direction?
Solution: Let $\vec{v}_{m}$ and $\vec{v}_{r}$ be velocities of the man and the river current w.r.t. the ground. The velocity of the man in still water is equal to the relative velocity of the man w.r.t. water i.e., \begin{align} \vec{v}_{m/r}=\vec{v}_m-\vec{v}_r. \nonumber \end{align} Let $\vec{v}_{m/r}$ and $\vec{v}_{m}$ make angle $\alpha$ and $\theta$ with the north direction. Let $d$ be the width of the river. The time taken by the man to cross the river is given by \begin{align} t&=\frac{\text{width of the river}}{\text{component of man velocity along north}} \nonumber\\ &=\frac{d}{|\vec{v}_m|\cos\theta}\nonumber\\ &=\frac{d}{|\vec{v}_{m/r}|\cos\alpha}. \\ &\qquad {(\because \mathrm{PQ}=t|\vec{v}_m|\cos\theta=t|\vec{v}_{m/r}|\cos\alpha).} \end{align} From above equation, $t$ is minimum when denominator is maximum. Given, $|\vec{v}_{m/r}|=10 \,\mathrm{metre/min}$, a constant. Thus, $t$ become minimum when $\cos\alpha =1$ i.e., $\alpha=0$. Hence, the man takes the shortest time when he swims perpendicular to the river velocity i.e., towards north.
Problem: A boat must get from point A to point B on the opposite bank of the river moving along a straight line AB that makes 120 degree angle with the flow direction. If distance AB is 2.5 km, the speed of boat in still water is 7 km/hr and the speed of the river current is 3 km/hr, then the minimum travel time of the boat is?
Solution: The speed of the river current is $v_r=3\;\mathrm{km/hr}$ and speed of the boat relative to the water is $v_{b/r}=7\;\mathrm{km/hr}$. The velocity of the boat relative to the ground should be along the line AB. Let $\vec{v}_{b/r}$ makes an angle $\theta$ with AB. \begin{align} v_{b/r}\cos(30+\theta)&=v_b\cos30\\ v_{b/r}\sin(30+\theta)&=v_r+v_b\sin30 \end{align} Substitute values, square and add to get \begin{align} 49=v_b^2+3 v_b +9. \end{align} Solve to get $v_b=5\;\mathrm{km/hr}$. Thus, time taken to travel a distance $\mathrm{AB}=2.5\; \mathrm{km}$ is $t=\mathrm{AB}/v_b=0.5\;\mathrm{hr}=30\;\mathrm{min}$.
Question: To cross a river in minimum time, your speed relative to the water should be perpendicular to the river flow.
Minimum Drift
Problem: A boat moves relative to water with a velocity 10 km/hr. The velocity of river flow is 5 km/hr. At what angle to the stream direction must the boat move to minimize drifting?
Solution: The drift is zero if boat reaches directly opposite point. To do so, the velocity of boat relative to the ground ($\vec{v}_b$) should be perpendicular to the flow velocity ($\vec{v}_w$). This can happen only if the speed of boat in still water ($\vec{v}_{b/w}$) is greater than flow speed, which is true in this problem.
Let $\vec{v}_{b/w}$ makes an angle $\theta$ with $\vec{v}_w=5\; \mathrm{km/hr}\;\hat\imath$. The relative velocity formula gives $\vec{v}_b=\vec{v}_{b/w}+\vec{v}_w$. Thus, $10\cos\theta=v_b$ and $10\sin\theta=5$ which gives $\theta=30$ degree.
Problem: A boat moves relative to water with a velocity 5 km/hr. The velocity of river flow is 10 km/hr. At what angle to the stream direction must the boat move to minimize drifting?
Solution: The speed of boat in still water ($v_{b/w}=5\;\mathrm{km/hr}$) is less than the flow speed ($v_{b}=10\;\mathrm{km/hr}$). The boat cannot cross the river to an exactly opposite point. In this case, the drift is minimum if $\vec{v}_{b}=\vec{v}_{b/w}+\vec{v}_w$ is perpendicular to $\vec{v}_{b/w}$. The angle made by $\vec{v}_{b/w}$ with $\vec{v}_w$ is given by \begin{align} \theta=\cos^{-1}-\frac{v_{b/w}}{v_w}=\cos^{-1}-\frac{1}{2}=150\;\mathrm{degree}. \end{align}
Exercise: A boat must get from point A to point B on the opposite bank of the river. The distances BC and AC are equal. The speed of river water is $v_w$. What is the minimum speed of the boat relative to the water?
Exercise: A man can swim with a speed of 4.0 km/h in still water. How long does he take to cross a river 1.0 km wide if the river flows steadily at 3.0 km/h and he makes his strokes normal to the river current? How far down the river does he go when he reaches the other bank?
Exercise: A boat is moving across a river whose water flows with a velocity $\vec{v}_w$. The velocity of the boat relative to the water is $\vec{v}_{b/w}$ and it makes an angle $\alpha$ to a line perpendicular to $\vec{v}_w$. What is the angle made by the velocity of the boat relative to the ground $\vec{v}_b$ with the line perpendicular to $\vec{v}_w$?
Practice Problems
- Two swimmers leave point A on one bank of the river to reach point B lying right across on the other bank. One of them crosses the river along the straight line AB while the other swims at right angles to the stream and then walks the distance that he has been carried away by the stream to get to point B. What was the velocity u of f his walking if both swimmers reached the destination simultaneously? The stream velocity v, = 2.0 km/hour and the velocity if of each swimmer with respect to water equals 2.5 km/hr.
- The width of the river is 1 km and velocity of river water is 5 km/hr. There are two boats at the same point on the bank. The velocity of each boat in still water is 10 km/hr. One of the boat starts its journey along the bank, it travels a distance 1 km upstream, and return back. Simultaneously, another boat starts its journey to the other bank by shortest path and returns back. Which of the boat returns to the starting point first?
- From a point A on a bank of a channel with still waters a person must get to a point B on the opposite bank. All the distances are shown in the figure. The person uses a boat to travel across the channel and then walks along the banks to point B. The velocity of the boat is $v_1$ and the velocity of walking person is $v_2$. Prove that the fastest way for the person to get from A to B is to select the angles $\alpha_1$ and $\alpha_2$ in such a manner that $\sin\alpha_1/\sin\alpha_2=v_1/v_2$.
